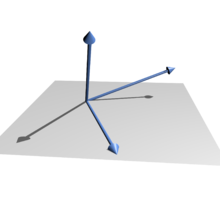
선형독립 Linearly Independent vs 선형종속 Linearly Dependent
위키백과에 따르면
In the theory of vector spaces, a set of vectors is said to be linearly dependent if at least one of the vectors in the set can be defined as a linear combination of the others if no vector in the set can be written in this way, then the vectors are said to be linearly independent.


'선형대수 | 해석학 | 통계학' 카테고리의 다른 글
| [통계학] Transformations and Expectations (0) | 2022.03.26 |
|---|---|
| [통계학] Probability Theory (0) | 2022.03.23 |
| [기초해석학] 실수의 특성 (0) | 2022.02.26 |
| [선형대수/머신러닝] 머신러닝에 기초인 선형대수 정리 (0) | 2021.08.31 |
| [선형대수/Linear Algebra] Rank(계수) 개념 정리 (0) | 2021.04.01 |